Last week I showed how, based on the Elliott Wave Principle (EWP), the S&P 500 and Dow Jones Industrial Average could have put in a multi-year top. (See here and here, respectively).
Besides, based on prior larger degree 4th waves and the EWP rules, I was able to logically deduct a highly likely path forward for the multi-year bear market the US major indexes could be in. Thus, if the SPX and DOW are in such a bear market, it is also logical to assume the NASDAQ Composite is in such a bear market. In this article, I use the NASDAQ’s yearly-resolution chart to show how this can be the case. See figure 1 below.
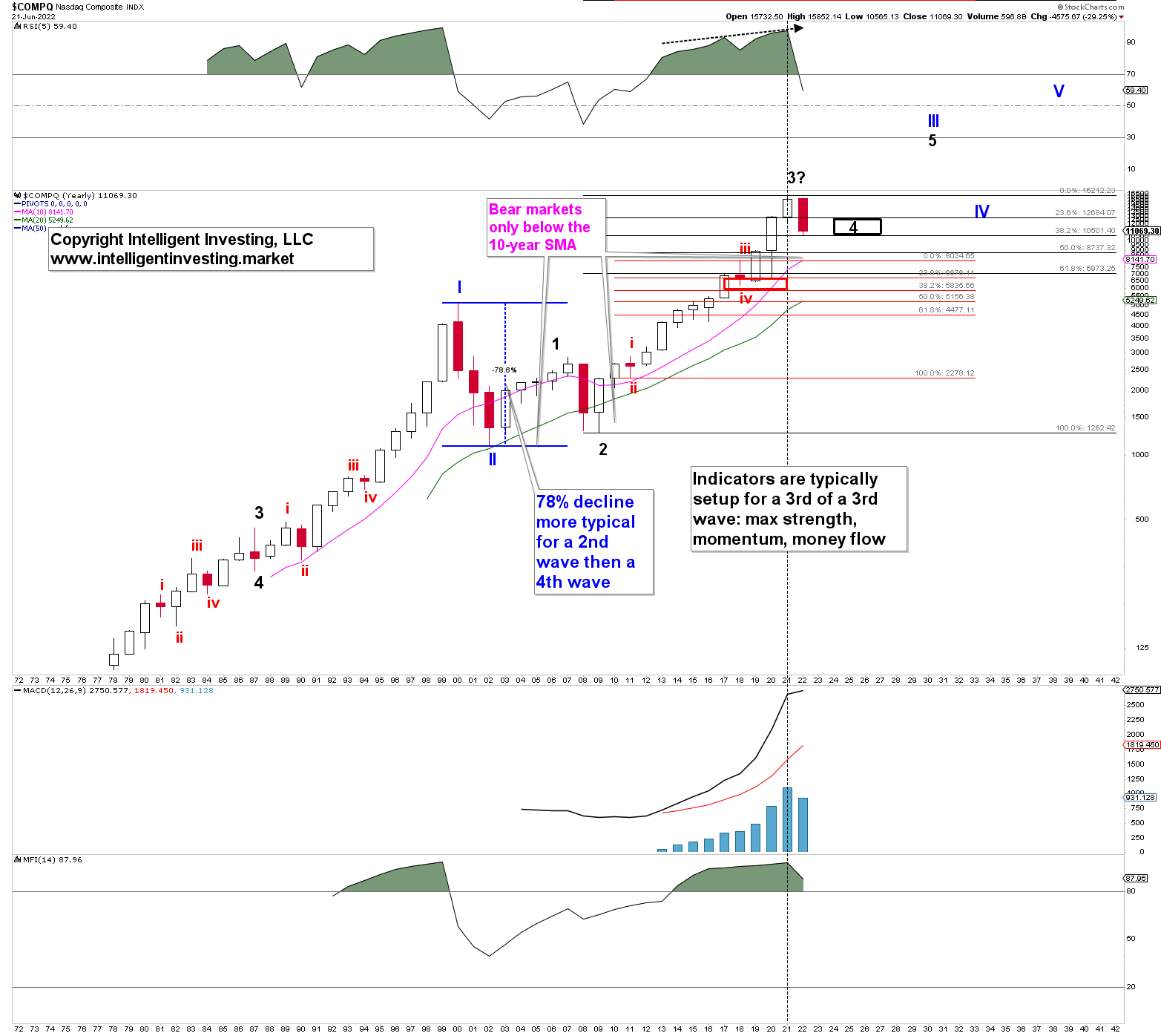
Figure 1. NASDAQ yearly candlestick chart with detailed EWP count. Note the log scale.
Down Years Are Corrective Years
This article will not do any price forecasts but rather show how counting down years as corrective years leads to concluding that the NASDAQ has possibly completed (black) major-3 of (blue) Primary-III. Thus, similar to the SPX and DOW, a larger degree 4th wave (of the 2007-2009) bear market level should now be underway.
This method can only confirm that thesis when the index closes lower for the year. Then the index has completed clean, clear five (red) waves up from the 2009 low. Hence, it will take six more months, but for now, this looks very feasibly, considering the index has now declined for five out of the last six months. And forewarned is forearmed.
The 78% haircut during the Dotcom bubble burst is typical for a 2nd wave (fast and deep), while the yearly (!) technical indicators confirm the presented EWP. Although the index has already reached the ideal downside target for this 4th wave (the 38.20% retrace of the entire rally that started in 2009), there’s no guarantee that level is it. In fact, the index can even retrace to the March 2020 lows over time.
However, as shown for the S&P 500 and DOW, the path to get there will likely be complex, thus hard to forecast, and long-drawn.
But again, this is not a method to make price predictions; it is a method to track the much larger EWP waves in the index--and every other index, as a matter of fact.
