Recently, 10-year real yields went to new all-time lows. Right now, they’re at -0.96%. What that means is that, if you buy TIPS, you’re locking in a loss of about 1% of your purchasing power, per year, over the next decade. If inflation goes up 2%, TIPS will return about 1%. If inflation goes up 8%, TIPS will return 7%. And so on.
With that reality, I’ve recently seen lamentations that TIPS are too expensive—who in the world would buy these real yields?!?
The answer, of course, is everybody. Indeed, if you can figure out a way to buy an asset without locking in the fundamental reality that the real risk-free rate is -1%, please let me know.
Because when you buy a nominal Treasury bond, you are buying them at a nominal interest rate that reflects a -1% real interest rate along with an expectation of a certain level of inflation. The whole point of the Fisher equation is that a nominal yield consists of (a) the real cost of money, and (b) compensation for the expected deterioration in the value of that money over time—expected inflation.[1] So look, if you buy nominal yields, you’re also getting that -1% real yield…it’s just lumped in with something else.
Well golly, then we should go to a corporate bond! Yields there are higher, so that must mean real yields are higher, right? Nope: the corporate yield is the real yield, plus inflation compensation, plus default risk compensation. Your yield is higher because you’re taking more (different) risks, but the underlying compensation you’re receiving for the cost of money is still -1%.
Commodities! Nope. Expected commodity index returns consist of expected collateral return, plus (depending how you count it) spot return and roll return. But that collateral return is just a fixed-income component…see above.
Equities, of course, have better expected returns over time not because they are somehow inherently better, but because buyers of equities earn a premium for taking on the extra risk of common equities—cleverly called the equity risk premium—over a risk-free investment.
In fact, the expected returns for all long positions in investments consist of the same basic things: a real return for the use of your money, and a premium for any risk you are taking over and above a riskless investment (the riskless investment being, we know, an inflation-linked bond and not a nominal bond). This is the whole point of the Capital Asset Pricing Model; this understanding is what gives us the Security Market Line, although it’s usually drawn incorrectly with T-bills as the risk-free asset. Here is the current market line we calculate, using our own models and with just a best-fit line in there showing the relationship between risk and return.
Not that long ago, that entire line was shifted higher more or less in parallel as real interest rates were higher along with the expected returns to every asset class:
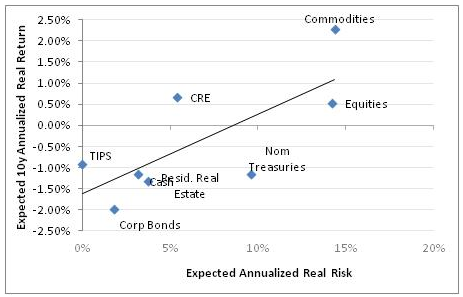
So why am I mentioning this? Because I have been hearing a lot recently about how people are buying stocks because TINA (There Is No Alternative) when yields are this low.
But if the capital asset pricing model means anything, that is poor reasoning: your return to equity investment incorporates the expected real return to a riskless asset. There is an alternative to equities and equity risk; what there’s no alternative to is the level of real rates. The expected real return from here for equities is exceptionally poor—but, to be fair, so are the expected real returns from all other asset classes, and for some of the same reasons.
This is a consequence, of course, of the massive amount of cash in the system. Naturally, the more cash there is, then the worse the real returns to cash because a borrower doesn’t need to compensate you as much for the use of your money when there’s a near-unlimited amount of money out there. And the worse the real returns to cash, the worse the real returns to everything else.
You can’t avoid it—it’s everywhere. I don’t know if it’s the new normal, but it is the normal for now.
[1] Unhelpfully, the Fisher equation also notes that there is an additional term in the nominal yield, which represents compensation being taken on by the nominal bondholder for bearing the volatility in the real outcome. But it isn’t clear why the lender, and not the borrower, ought to be compensated for that volatility…the borrower of course also faces volatility in real outcomes. In any event, it can’t be independently measured so we usually just lump that in with the premium for expected inflation.
