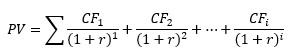When I started working in the financial markets, bond traders were the cool kids. The equity guys drove Maseratis and acted like buffoons, but the bond guys drove sensible style like Mercedes and cared about things like deficits and credit. The authoritative word on this subject came from the book Liar’s Poker by Michael Lewis, about 1980s Salomon Brothers, where the trainees dreaded being assigned to do Equities in Dallas.
Back then, equities guys worried about earnings, the quality of management and the balance sheet, and the really boring ones worried about a margin of safety and investing at the right price. That seems Victorian now, but I guess so does the idea that sober institutions should only own bonds.
Down the list of concerns, but still on it, were interest rates. Ol’ Marty Zweig used to have a commercial in which he said “if you can spot meaningful changes (not just zig-zags) in interest rates and momentum, you’ll be mostly in stocks during major advances and out during major declines.” The reason that interest rates matter at all to a stock jockey is that the present value of any series of cash flows, such as dividends, depends on the interest rate used to discount those cash flows.
In general, if the discount curve (yield curve) is flat, then the present value (PV) of a series of cash flows (CF) is the sum of the present values of each cash flow:
…where r is the interest rate.
As a special case, if all of the cash flows are equal and go on forever, then we have a perpetuity where PV = CF/r. Note also that if all of the cash flows have the same real value and are only adjusted for inflation, and the denominator is a real interest rate, then you get the same answer to the perpetuity problem.[1]
I should say right now that the point of this article is not to go into the derivation of the Gordon Growth Model, or argue about how you should price something where the growth rate is above the discount rate, or how you treat negative rates in a way that doesn’t make one’s head explode. The point of this article is merely to demonstrate how the sensitivity of that present value to the numerator and the denominator changes when interest rates change.
The sensitivity to the numerator is easy. PV is linear with respect to CF. That is, if the cash flow increases $1 per period, then the present value of the whole series increases the same amount regardless of whether we are increasing from $2 to $3 or $200 to $201. In the table below, the left two columns represent the value of a $5 perpetuity versus a $6 perpetuity at various interest rates; the right two columns represent the value of a $101 perpetuity versus a $102 perpetuity. You can see that in each case, the value of the perpetuity increases the same amount going left to right in the green columns as it does going left to right in the blue columns. For example, if the interest rate is 5%, then an increase in $1 increases the total value by $20 whether it’s from $5 to $6 or $100 to $101.
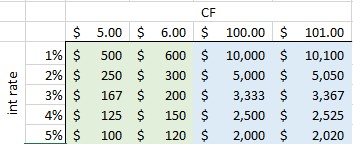
However, the effect of the same-sized movement in the denominator is very different. We call this sensitivity to interest rates duration, and in one of its forms that sensitivity is defined as the change in the price for a 1% change in the yield.[2]
Moving from 1% to 2% cuts the value of the annuity (in every case) by 50%, but moving from 4% to 5% cuts the value by only 20%.
What this means is that if interest rates are low, you care a great deal about the interest rate. Any change to your numerator is easily wiped out by a small change in the interest rate you are discounting at. But when interest rates are higher, this is less important and you can focus more on the numerator. Of course, in this case we are assuming the numerator does not change, but suppose it does? The importance of a change in the numerator depends not on the numerator, but on the denominator. And for a given numerator, any change in the denominator gets more important at low rates.
So, where am I going with this?
Let’s think about the stock market. For many years now, the stock market has acted as if what the Fed does is far more important than what the businesses themselves do. And you know what? Investors were probably being rational by doing so. At low interest rates, the change in the discount rate was far more important – especially for companies that don’t pay dividends, so they’re valued on some future harvest far in the future – than changes in company fortunes.
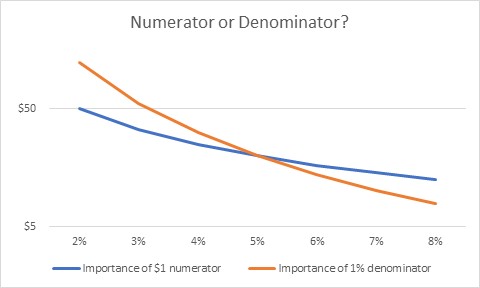
However, as interest rates rise this becomes less true. As interest rates rise, investors should start to care more and more about company developments. I don’t know that there is any magic about the 5% crossover that I have in that chart (the y-axis, by the way, is logarithmic because otherwise the orange line gets vertical as we get to the left edge!).
But it suggests to me that stock-picking when interest rates are low is probably pointless, while stock-picking when interest rates are higher is probably fairly valuable. What does an earnings miss mean when interest rates are at zero? Much less than missing on the Fed call. But at 5%, the earnings miss is a big deal.
Perhaps this article, then, is mistitled. It isn’t that we are all bond traders now. It’s that, until recently, we all were bond traders…but this is less and less true.
And it is more and more true that forecasts of weak earnings growth for this year and next – are much more important than the same forecasts would have been, two years ago.
But the bond traders are still the cool kids.
***
- I should also note that r > 0, which is something we never had to say in the past. In nominal space, anyway, it would be an absurdity to have a perpetually negative interest rate, implying that future cash flows are worth more and more…and the perpetuity has infinite value.
- Purists will note that the duration at 2% is neither the change in value from 1% to 2% nor from 2% to 3%, but rather the instantaneous change at 2%, scaled by 100bps. But again, I’m not trying to get to fine bond math here and just trying to make a bigger point.